Question

A tennis ball is thrown straight up into the air with an initial velocity of 12.7 m/s. How high does the tennis ball travel?

Answer 1

8.22m

Step-by-step explanation:

As interpreted from this problem, the angle of launch is 90 degrees as it is thrown straight up. Given an angle launch of 90°, upward initial velocity of 12.7m/s, and initial height of 0m. A much simpler equation can be used to find the maximum height.

`h_(max) = h_ {0} + \frac{{v_0}^(2) }{2g}`

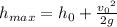
this means that the max height is equal to the initial height plus the initial velocity squared divided by 2 times the acceleration of earth's gravity(≈9.8m/s^2 or 10m/s^2 depending on how you fix it).

when substituted into the equation, you should get.

(9.8m/s^2)

hmax = 0 + ((12.7)^2)/(2*9.8)

hmax = 161.29/19.6 ≈ 8.22

hmax = 8.22m