Question

Another 2 needed. 50 points!

Please show work, Thanks! :)

Answer 1

Coordinate geometry is one of the most important and exciting ideas of mathematics. In particular it is central to the mathematics students meet at school. It provides a connection between algebra and geometry through graphs of lines and curves. This enables geometric problems to be solved algebraically and provides geometric insights into algebra.

The invention of calculus was an extremely important development in mathematics that enabled mathematicians and physicists to model the real world in ways that was previously impossible. It brought together nearly all of algebra and geometry using the coordinate plane. The invention of calculus depended on the development of coordinate geometry.

Explanation:

Answer 2

1) B

2) C

Explanation:

Question 1)

We have the function:

`y=2x^2+24x-16`

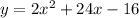
Note that this is in the standard quadratic form:

`y=ax^2+bx+c`

Unfortunately, since this isn't in vertex form, we need to do a bit more work for our vertex.

Remember that we can find our vertex using the following formulas:

`x=-(b)/(2a),\ y=f(-(b)/(2a))`

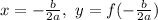
Let's label our coefficients. Our a is 2, b is 24, and c is -16.

Let's find our vertex. Substitute 24 for b and 2 for a. This yields:

`x=-(24)/(2(2))`

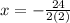
Multiply:

`x=-(24)/(4)`

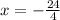
Divide:

`x=-6`

So, the x-coordinate of our vertex is -6.

Now, to find the y-coordinate, we simply need to substitute x for our equation. We have:

`y=2x^2+24x-16`

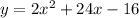
Substitute -6 for x:

`y=2(-6)^2+24(-6)-16`

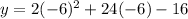
Evaluate. Square:

`y=2(36)+24(-6)-16`

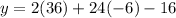
Multiply:

`y=72-144-16`

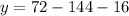
Subtract. So, the y-coordinate of our vertex is:

`y=-88`

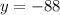
So, our vertex point is (-6, -88).

Remember that the axis of symmetry is the same as the x-coordinate of our vertex. So, our axis of symmetry is at x=-6.

Therefore, our answer is B.

Question 2)

We have the equation:

`y=-2x^2+8x-20`

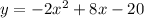
Again, we can use the above formula. Let's label of coefficients.

Our a is -2, b is 8, and c is -20.

So, let's find the x-coordinate of our vertex:

`x=-(b)/(2a)`

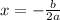
Substitute 8 for b and -2 for a:

`x=-(8)/(2(-2))`

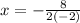
Multiply:

`x=-(8)/(-4)`

Divide. The negatives cancel. So, our x-coordinate is:

`x=2`

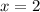
Now, substitute this back into the equation to find the y-coordinate:

`y=-2(2)^2+8(2)-20`

Square:

`y=-2(4)+8(2)-20`

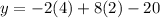
Multiply:

`y=-8+16-20`

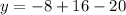
Add:

`y=-12`

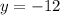
Therefore, our vertex is (2, -12).

And the axis of symmetry is at x=2.

Our answer is C.

And we're done!