Question

Write an equation of the line passing through each of the following pairs of points.

(5, 6), (-1,4)

Answer 1

`y=(1)/(3)+(13)/(3)`

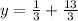
Explanation:

We want to write the equation of a line that passes through (5, 6) and (-1, 4).

First, let's find our slope. We can use the slope formula:

`m=(y_2-y_1)/(x_2-x_1)`

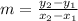
Let's let (5, 6) be (x₁, y₁) and let's let (-1, 4) be (x₂, y₂). So, our slope is:

`m=(4-6)/(-1-5)`

Subtract:

`m=-2/-6`

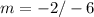
Reduce:

`m=1/3`

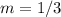
So, our slope is 1/3.

Now, we can use the point-slope form, which is:

`y-y_1=m(x-x_1)`

For consistency, let's let (5, 6) be (x₁, y₁). We will also substitute 1/3 for m. So:

`y-6=(1)/(3)(x-5)`

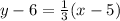
Distribute:

`y-6=(1)/(3)x-(5)/(3)`

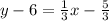
Add 6 to both sides:

`y=(1)/(3)x-(5)/(3)+(18)/(3)`

Add:

`y=(1)/(3)+(13)/(3)`

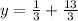
And we're done!