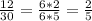Question

Eric has a riddle: "I am thinking of a fraction that is equivalent to

2
5
and the numerator is 18 less than the denominator."

What is Eric's fraction

Answer 1

Answer:
`(12)/(30)`

This is the same as writing 12/30

===========================================================

Step-by-step explanation:

d = value of the denominator

d-18 = value of the numerator, since it's 18 less than the denominator

The fraction Eric is thinking of is
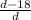`(d-18)/(d)` where d cannot be zero. This fraction is equivalent to
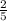`(2)/(5)` so we'll set the two fractions equal to one another and solve for d.

`(d-18)/(d) = (2)/(5)\\\\5(d-18) = 2d \ \ \text{ ... cross multiply}\\\\5d-90 = 2d\\\\5d-2d = 90\\\\3d = 90\\\\d = (90)/(3)\\\\d = 30\\\\`

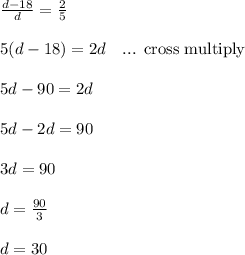
The denominator is d = 30 and the numerator is d-18 = 30-18 = 12.

The unreduced fraction Eric is thinking of is
`\Large (12)/(30)`.

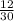To verify the answer, notice that:

`(12)/(30) = (6*2)/(6*5) = (2)/(5)`