Answer:
n² ≤ 2ⁿ , ∀ n ≥ 4
Explanation:
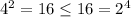
Then ,For n = 4 the inequality is true.
For n ≥ 4 , suppose n² ≤ 2ⁿ and prove that (n+1)² ≤ 2ⁿ⁺¹
(n+1)² = n² + 2n + 1
Since n² ≤ 2ⁿ (according to the hypothesis) and we know that 2n + 1 ≤ 2ⁿ
Then
(n+1)² = n² + 2n + 1 ≤ 2ⁿ + 2ⁿ
Then
(n+1)² ≤ 2ⁿ + 2ⁿ
Then
(n+1)² ≤ 2×2ⁿ
Then
(n+1)² ≤ 2ⁿ⁺¹
Conclusion:
n² ≤ 2ⁿ , ∀ n ≥ 4