Answer:
The answer is (C)
Explanation:
Let us multiply the two trinomials and simplify the product to find the correct choice.
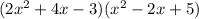
To find the product multiply each term in the first bracket by the second bracket, then add the like terms
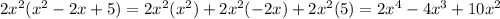

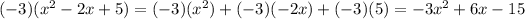
Now let us add the like terms.

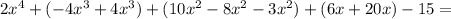
Simplify each term
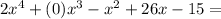

By comparing it with the choices
The answer is (C)