Question

In a test of a new UV-curable polymeric material (with thermal conductivity 0.15 W/(m K)) for dental prostheses, a 2.8 mm thick slab is subjected to monochromatic light with wavelength 313 nm, which is absorbed by the polymeric material according to the Beer Lambert Law of exponential attenuation. The material's surface is exposed to light at z = 0 , the rate at which light is absorbed (with dimensions of photons per unit volume per unit time) is 0 z I e γ γ − , where 0 I is the incident photon flux (with dimensions of photons per unit area per unit time), and 4 1 γ 3 10 m− − = × . (This approximation neglects the temporal decrease in absorption, among other things.) We also assume that all absorbed radiation is converted to thermal energy. (This neglects thermochemical change in the material.) If the two surfaces of the polymeric material (at z = 0 and z = 2.8 mm ) are held at 38°C, what is the largest allowable value of 0 I so that, at steady state, the maximum temperature in the polymeric material does not exceed 44°C

Answer 1

The largest allowable Io = 5.863 * 10^26 photons

Step-by-step explanation:

thickness of slab = 2.8 mm

First we express the energy of each photon

`E = (hc)/(wavelength)`

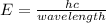
wavelength = 313 nm

attached below is the detailed solution on how i arrived at the answer above

note : we use 2 in the integral because the slab is exposed to light from two different direction