Question

2. Suppose a new standardized test is given to 100 randomly selected third-grade students in New Jersey. The sample average score Y on the test is 58 points and the sample standard deviation sY is 8 points. a. The authors plan to administer the test to all third-grade students in New Jersey. Construct a 95% con dence interval for the mean score of all New Jersey third graders. b. Suppose the same test is given to 200 randomly selected third graders from Iowa, producing a sample average of 62 points and sample standard deviation of 11 points. Construct a 90% con dence interval for the di erence in mean scores between Iowa and New Jersey. c. Can you conclude with a high degree of con dence that the population means for Iowa and New Jersey students are di erent

Answer 1

(a) A 95% confidence interval for the mean score of all New Jersey third graders is [56.41, 59.59] .

(b) A 90% confidence interval for the difference in mean scores between Iowa and New Jersey is [3.363, 4.637] .

(c) Yes, we are 90% confident that the population means for Iowa and New Jersey students are different.

Explanation:

We are given that a new standardized test is given to 100 randomly selected third-grade students in New Jersey. The sample average score Y on the test is 58 points and the sample standard deviation sY is 8 points.

(a) Firstly, the pivotal quantity for finding the confidence interval for the population mean is given by;

P.Q. =
`(\bar X-\mu)/((s)/(√(n) ) )` ~
`t_n_-_1`

where,
`\bar X` = sample average score = 58 points

s = sample standard deviation = 8 points

n = sample of third-grade students = 100

`\mu` = population mean score of all New Jersey third graders

Here for constructing a 95% confidence interval we have used a One-sample t-test statistics because we don't know about population standard deviation.

So, a 95% confidence interval for the population mean,
`\mu` is;

P(-1.987 <
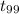`t_9_9` < 1.987) = 0.95 {As the critical value of t at 99 degrees of

freedom are -1.987 & 1.987 with P = 2.5%} P(-1.987 <
`(\bar X-\mu)/((s)/(√(n) ) )` < 1.987) = 0.95

P(
`-1.987 * {(s)/(√(n) ) }` <
`{\bar X-\mu}` <
`1.987 * {(s)/(√(n) ) }` ) = 0.95

P(
`\bar X-1.987 * {(s)/(√(n) ) }` <
`\mu` <
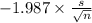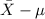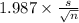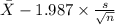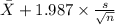`\bar X+1.987 * {(s)/(√(n) ) }` ) = 0.95

95% confidence interval for
`\mu` = [
`\bar X-1.987 * {(s)/(√(n) ) }` ,
`\bar X+1.987 * {(s)/(√(n) ) }` ]

= [
`58-1.987 * {(8)/(√(100) ) }` ,
`58+1.987 * {(8)/(√(100) ) }` ]

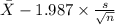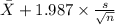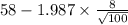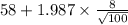= [56.41, 59.59]

Therefore, a 95% confidence interval for the mean score of all New Jersey third graders is [56.41, 59.59] .

Now, the same test is given to 200 randomly selected third graders from Iowa, producing a sample average of 62 points and a sample standard deviation of 11 points.

(b) Firstly, the pivotal quantity for finding the confidence interval for the difference in population mean is given by;

P.Q. =
`\frac{(\bar X_1-\bar X_2)-(\mu_1-\mu_2)}{s_p * \sqrt{(1)/(n_1)+(1)/(n_2) } }` ~
`t__n_1_+_n_2_-_2`

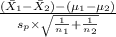where,
`s_p = \sqrt{((n_1-1)* s_1 + (n_2-1)* s_2)/(n_1+n_2-2) }`

=
`\sqrt{((200-1)* 11 + (100-1)* 8)/(200+100-2) }` = 3.163

Here for constructing a 90% confidence interval we have used a two-sample t-test statistics because we don't know about population standard deviations.

So, a 90% confidence interval for the difference in two population means, (
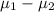`\mu_1-\mu_2`) is;

P(-1.645 <
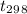`t_2_9_8` < 1.645) = 0.90 {As the critical value of t at 298 degrees of

freedom are -1.645 & 1.645 with P = 5%} P(-1.645 <
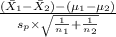`\frac{(\bar X_1-\bar X_2)-(\mu_1-\mu_2)}{s_p * \sqrt{(1)/(n_1)+(1)/(n_2) } }` < 1.645) = 0.90

90% confidence interval for (
`\mu_1-\mu_2`) = [
`{(\bar X_1-\bar X_2) -1.645* {s_p * \sqrt{(1)/(n_1)+(1)/(n_2) } }` ,
`{(\bar X_1-\bar X_2) +1.645* {s_p * \sqrt{(1)/(n_1)+(1)/(n_2) } }` ]

= [
`{(62-58) -1.645* {3.163 * \sqrt{(1)/(200)+(1)/(100) } }` ,
`{(62-58) +1.645* {3.163 * \sqrt{(1)/(200)+(1)/(100) } }` ]

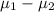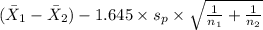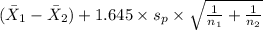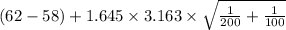= [3.363, 4.637]

Therefore, a 90% confidence interval for the difference in mean scores between Iowa and New Jersey is [3.363, 4.637] .

(c) Yes, we are 90% confident that the population means for Iowa and New Jersey students are different because in the above interval 0 is not included.