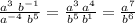Answer:
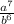 (second option in your list)
(second option in your list)
Explanation:
Notice that every base with negative exponent can be written with positive exponent on the opposite side of the fraction line, therefore:
The a to the exponent "-4" in the denominator can be written as a to the exponent 4 in the numerator, thus giving two bases a in the numerator, one with exponent 3 and another one with exponent 4 which combined with the rule of product of numbers with same base, leads to the addition ofthe exponents: a to the power 7 in the numerator.
We do something similar with the base b (which in the numerator appears with a "-1" in the exponent), we write it in the denominator with exponent "+1".
Therefore we end up with the following: