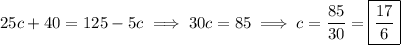It sounds like you're asked to find c such that f(x), defined by
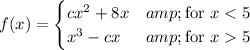
is continuous at x = 5.
With the strict inequalities given in the definition, this is not possible. So you probably meant to use ≤ or ≥ in one of the pieces of the definition.
In order for f(x) to be continuous at x = 5, the limit from either side as x approaches 5 must be the same.
We have
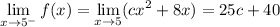
and
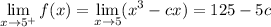
Then