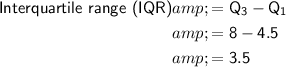Question

The box plot below represents the lengths, in inches, of fish a group of friends caught.

What is the interquartile range of the data?

Answer 1

IQR = 3.5

Explanation:

Explanation of the given box plot:

• The closed circles at either end are the minimum and maximum
• The middle vertical line of the box is the median
• The left side of the box is the first quartile
• The right side of the box is the third quartile

Therefore, the Five-number summary of the given box plot is:

• Minimum = 3
• First quartile (Q₁) = 4.5
• Median (Q₂) = 6
• Third quartile (Q₃) = 8
• Maximum = 13

`\begin{aligned}\textsf{Interquartile range (IQR)} & = \sf Q_3-Q_1\\& = \sf 8 - 4.5\\&= \sf 3.5\end{aligned}`

Answer 2

• 3.5

Explanation:

According to the box plot we have

• Q1 = the 25% mark of data = 4.5 and
• Q3 = the 75% mark of data = 8

The IQR is the difference of the two numbers above

• IQR = Q3 - Q1 = 8 - 4.5 = 3.5