Answer:
Perpendicular
Explanation:
Parallel lines mean lines that have same slope but since both equations have different slopes which you can check by looking at m-value in y = mx + b. In this case m1 or first slope is -4/3 and m2 or second slope is 3/4.
Perpendicular means that both lines are reciprocal to each other. This means the perpendicular condition is or satisfies
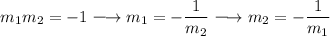
We have m1 = -4/3 and m2 = 3/4.
Therefore, -4/3 * 3/4 = -1 thus both lines are perpendicular to each other as it satisfies m1m2 = -1 condition.