Question

What operation makes the statement true; 18 __ (-20) =-2

Answer 1

18-20=-2

Explanation:

18-20=-2

18+(-20)=-2

Answer 2

`\huge\boxed{\text{+}}`

Explanation:

To make this statement true, we want to make it so that when -20 is "something'd" to 18, we get -2.

Let's try all the common operators first - add, subtract, multiply, divide.

Let's try add.

If we put a plus sign there:

`18 + (-20) = -2`

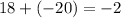
Adding a negative is the same as subtracting a positive.

`18-20=-2`

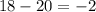
This is true! 18 - 20 is indeed -2. This means + is the right operator.

For fun, let me briefly go over what happens with all the other operators.

Subtract:

`18 - (-20) = -2\\\\18+20=-2\\\\28 \\eq -2`

Multiply:

`18 \cdot (-20) = -2\\\\-360 \\eq -2`

Divide:

`18 / (-20) = -2\\\\-0.9 \\eq -2`

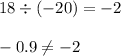
Hope this helped!