Answer:
The electric field at E at point P is represented by
![\vec E_(tot) = -3106.063\,\hat{i}+9694.512\,\hat{j}+5436.229\,\hat{k}\,\,\left[(N)/(C) \right]](https://img.qammunity.org/2021/formulas/physics/college/eeozf94m7uwzvhwk0p8ky17odklb1wykmk.png) .
.
Step-by-step explanation:
The definition of the electric field is represented by the following differential equation:
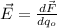
Where:
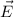 - Electric field, measured in newtons per coulomb.
- Electric field, measured in newtons per coulomb.
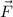 - Electrostatic force, measured in newtons.
- Electrostatic force, measured in newtons.
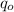 - Theoretical electric charge, measured in coulombs.
- Theoretical electric charge, measured in coulombs.
Electrostatic force is modelled by Coulomb's Law, which states that such force is directly proportional to electric current and inversely proportional to square distance. In this case, vectorial form of this law is:

Where:
 - Electrostatic constant, measured in newton-square meters per square coulomb.
- Electrostatic constant, measured in newton-square meters per square coulomb.
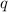 - Real electric charge, measured in coulombs.
- Real electric charge, measured in coulombs.
 - Magnitude of the vector position, measured in meters.
- Magnitude of the vector position, measured in meters.
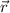 - Vector position, measured in meters.
- Vector position, measured in meters.
We get the electric field equation by deriving the equation above:
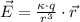
The total electric field is obtained by means of this concept and the Principle of Superposition as well:
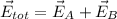
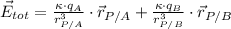
At first we determine each vector position and their magnitudes:
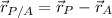
![\vec r_(P/A) = 0.15\,\hat{i}+0.20\,\hat{j}+0.50\,\hat{k}-0.10\,\hat{i}+0.10\,\hat{j}-0.10\,\hat{k}\,\,[m]](https://img.qammunity.org/2021/formulas/physics/college/2jtmtp70ifi6f1caid9l3prqj3bf4xr8jc.png)
![\vec r_(P/A) = 0.05\,\hat{i}+0.30\,\hat{j}+0.40\,\hat{k}\,\,[m]](https://img.qammunity.org/2021/formulas/physics/college/765hg60dds2x9vv7o4c2sideipjiktcd8y.png)
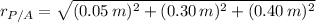
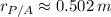
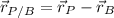
![\vec r_(P/B) = 0.15\,\hat{i}+0.20\,\hat{j}+0.50\,\hat{k}+0.10\,\hat{i}-0.15\,\hat{j}-0.05\,\hat{k}\,\,[m]](https://img.qammunity.org/2021/formulas/physics/college/jr1k4dzlcwqai35e05vxn5jfhx7nypt47u.png)
![\vec r_(P/B) = 0.25\,\hat{i}+0.05\,\hat{j}+0.45\,\hat{k}\,\,[m]](https://img.qammunity.org/2021/formulas/physics/college/asmfx6yu5cwo8meri4vfz6pexqs7rt6g3k.png)
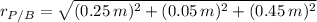
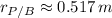
If we know that
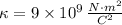 ,
,
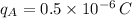 ,
,
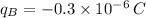 ,
,
![\vec r_(P/A) = 0.05\,\hat{i}+0.30\,\hat{j}+0.40\,\hat{k}\,\,[m]](https://img.qammunity.org/2021/formulas/physics/college/765hg60dds2x9vv7o4c2sideipjiktcd8y.png) ,
,
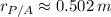 ,
,
![\vec r_(P/B) = 0.25\,\hat{i}+0.05\,\hat{j}+0.45\,\hat{k}\,\,[m]](https://img.qammunity.org/2021/formulas/physics/college/asmfx6yu5cwo8meri4vfz6pexqs7rt6g3k.png) and
and
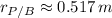 , then we get the electric field at point P.
, then we get the electric field at point P.
![\vec{E}_(tot) = \left[(\left(9* 10^(9)\,(N\cdot m^(2))/(C^(2)) \right)\cdot (0.5* 10^(-6)\,C))/((0.502\,m)^(3)) \right]\cdot (0.05\,\hat{i}+0.30\,\hat{j}+0.40\,\hat{k})\,\,[m] + \left[(\left(9* 10^(9)\,(N\cdot m^(2))/(C^(2)) \right)\cdot (-0.3* 10^(-6)\,C))/((0.517\,m)^(3)) \right]\cdot (0.25\,\hat{i}+0.05\,\hat{j}+0.45\,\hat{k})\,\,[m]](https://img.qammunity.org/2021/formulas/physics/college/xrmcjbbyqt0eg4csh5w64348m9cjrzfx1r.png)
![\vec E_(tot) = 1778.572\,\hat{i}+10671.439\,\hat{j}+14228.573\,\hat{k} - 4884.635\,\hat{i}-976.927\,\hat{j}-8792.344\,\hat{k}\,\,\left[(N)/(C) \right]](https://img.qammunity.org/2021/formulas/physics/college/am2wy6kxj07itw37lloqr6wdybwr2t31zx.png)
![\vec E_(tot) = -3106.063\,\hat{i}+9694.512\,\hat{j}+5436.229\,\hat{k}\,\,\left[(N)/(C) \right]](https://img.qammunity.org/2021/formulas/physics/college/eeozf94m7uwzvhwk0p8ky17odklb1wykmk.png)
The electric field at E at point P is represented by
![\vec E_(tot) = -3106.063\,\hat{i}+9694.512\,\hat{j}+5436.229\,\hat{k}\,\,\left[(N)/(C) \right]](https://img.qammunity.org/2021/formulas/physics/college/eeozf94m7uwzvhwk0p8ky17odklb1wykmk.png) .
.