Answer:
Explanation:
Initial length of the cardboard was y cm, and width was x cm.
The new dimensions are:
length = (y - 25) cm
width = (x - 15) cm
Area of the new rectangle = length x width
= (y - 25) x (x - 15)
= (y - 25) (x - 15)
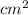
Perimeter = 2 ( length + width)
= 2 ((y - 25) + (x - 15))
= 2(y - 25) + 2(x - 15)
Therefore:
2(x + y) - 40 is neither of these
2(x - 15) + 2(y - 25) is the perimeter
(x - 15)(y - 25) is the area
(x - 25)(y - 15) is neither of these
2(x - 15)(y - 25) is neither of these
2x + 2y - 80 is the perimeter
xy - 375 is neither of these
x + y - 40 is neither of these