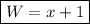A rectangle has an area of 6x + 6 cm2 and a length of 6 cm. What is the width of the rectangle? Enter your answer in the box. cm

Question

asked Nov 15, 2021 28.0k views

2 Answers

Josh Yeager · Answer 1 · 2021-11-21T16:30:33+0000

Answer:

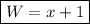
$\displaystyle \boxed{W=x+1}$

Explanation:

Area of a rectangle

A rectangle of length L and width W has an area of:

A = L*W

If the area and one of the dimensions are given, we can calculate the other dimension by solving the resulting equation.

The area of the given rectangle is 6x+6 square centimeters, and its length is 6 cm, thus we can establish the equation:

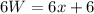
6W=6x+6

Solving for W:

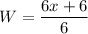
$\displaystyle W=(6x+6)/(6)$

The numerator 6x+6 can be factored as 6(x+1). Replacing in the equation:

$\displaystyle W=(6(x+1))/(6)$

Simplifying, the width of the rectangle in cm is:

$\displaystyle \boxed{W=x+1}$