Question

8. Given the following​ function, (a) find the​ vertex; (b) determine whether there is a maximum or a minimum​ value, and find the​ value; (c) find the​ range; and​ (d) find the intervals on which the function is increasing and the intervals on which the function is decreasing.

Answer 1

(a) (7,39/2)

(b) Maximum, 39/2

(c) y ≤ 39/2

(d) Increase when x < 7 and decrease when x > 7

Explanation:

Given the parabola:

`\displaystyle \large{f(x)=-(1)/(2)x^2+7x-5}`

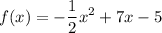
( A ) Find the vertex

In order to find the vertex, let’s use calculus for this one. Recall the power rules for differentiation:

Power Rules

`\displaystyle \large{f(x)=x^n \to f'(x)=nx^(n-1)}\\\\\displaystyle \large{f(x)=kx^n \to f'(x)=knx^(n-1) \quad \tt{(k \ \ is \ \ constant)}}\\\\\displaystyle \large{f(x)=k \to f'(x)=0 \quad \tt{(k \ \ is \ \ constant)}}`

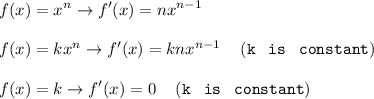
Derivative Definition

• Derivative f'(x) is a slope itself or rate of changes.

Derive the parabola:

`\displaystyle \large{f'(x)=-(1)/(2)(2)x^(2-1) + 7(1)x^(1-1) -0}\\\\\displaystyle \large{f'(x)=-x+7}`

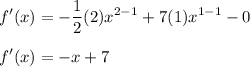
Since vertex has slope = 0 —> e.g f'(x) = 0:

`\displaystyle \large{0=-x+7}\\\\\displaystyle \large{-x=-7}\\\\\displaystyle \large{x=7}`

Substitute x = 7 in f(x):

`\displaystyle \large{f(7)=-(1)/(2)(7)^2+7(7)-5}\\\\\displaystyle \large{f(7)=-(1)/(2)(49)+49-5}\\\\\displaystyle \large{f(7)=-(49)/(2)+44}\\\\\displaystyle \large{f(7)=-(49)/(2)+(88)/(2)}\\\\\displaystyle \large{f(7)=(39)/(2)}`

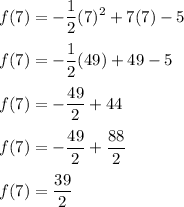
Therefore, the vertex is at (7,39/2)

( B ) Determine if max or min then find the value

Since the parabola opens downward then there only exists maximum value. The maximum value is the y-value of vertex at x-value of vertex. Henceforth:

• There is maximum value but no minimum value and the maximum value is 39/2 at x = 7.

( C ) Find range

For parabola, range is minimum value </≤ y </≤ maximum value. We know that parabola has maximum value of 39/2 but no minimum value so we can just ignore it then we’d have:

• y ≤ 39/2 is our range

[Note: < and > are for open-dot meaning the value will not be included —> e.g x > 4 means 4 isn’t included in.]

( D ) Find the interval when function is increasing and when it’s decreasing

For parabola, the function will increase only if f'(x) or slope > 0 and will decrease only f'(x) < 0.

We know, from part A that the derivative is:

`\displaystyle \large{f'(x)=-x+7}`

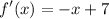
Therefore, when f'(x) > 0 —> e.g -x + 7 > 0:

`\displaystyle \large{-x+7 > 0}\\\\\displaystyle \large{-x > -7}\\\\\displaystyle \large{x < 7}`

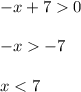
When f'(x) < 0 —> e.g -x + 7 < 0:

`\displaystyle \large{-x+7 < 0}\\\\\displaystyle \large{-x < -7}\\\\\displaystyle \large{x > 7}`

Therefore, the function will increase when x < 7 and will decrease when x > 7.

Answer 2

Explanation:

Parabola:

`\sf f(x) =(-1)/(2)x^2+7x - 5\\\\a = (-1)/(2) \ ; b = 7 \ ; \ c = -5`

`\sf x = (-b)/(2a)\\\\x =(-7)/(2*(-1)/(2))\\\\ = (-7)/(-1)`

x = 7

Now, to find the y-value substitute this in the equation

`\sf f(x) = (-1)/(2)*7^2+7*7-5\\\\ =(-49)/(2)+49-5\\\\ = (-49)/(2)+44\\\\ = (-49)/(2)+(88)/(2)\\\\= (39)/(2)`

`\boxed{Vertex(7 ,(39)/(2))}`

b) The value of a is negative. So, the parabola is open down words and the maximum value is given by the y-coordinate of the Vertex.

`\sf \boxed{Maximum \ value= (39)/(2)}`

`\sf c) Range = [(39)/(2), -infinity)`