Answer:
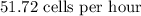
Explanation:
So, the function, P(t), represents the number of cells after t hours.
This means that the derivative, P'(t), represents the instantaneous rate of change (in cells per hour) at a certain point t.
C)
So, we are given that the quadratic curve of the trend is the function:

To find the instanteous rate of growth at t=5 hours, we must first differentiate the function. So, differentiate with respect to t:
![(d)/(dt)[P(t)]=(d)/(dt)[6.10t^2-9.28t+16.43]](https://img.qammunity.org/2021/formulas/mathematics/high-school/rxytc1oowx5v1aqb6lfls27ya449nstkrf.png)
Expand:
![P'(t)=(d)/(dt)[6.10t^2]+(d)/(dt)[-9.28t]+(d)/(dt)[16.43]](https://img.qammunity.org/2021/formulas/mathematics/high-school/nterxv15dgb0kz52xtmbalq3lmt02t73ia.png)
Move the constant to the front using the constant multiple rule. The derivative of a constant is 0. So:
![P'(t)=6.10(d)/(dt)[t^2]-9.28(d)/(dt)[t]](https://img.qammunity.org/2021/formulas/mathematics/high-school/tagl6tdg1ahhe2htsj995cyq0l6u4ge0n7.png)
Differentiate. Use the power rule:
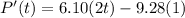
Simplify:
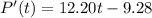
So, to find the instantaneous rate of growth at t=5, substitute 5 into our differentiated function:
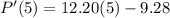
Multiply:
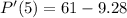
Subtract:
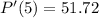
This tells us that at exactly t=5, the rate of growth is 51.72 cells per hour.
And we're done!