Answer:
m is parallel to n
Explanation:
Calculate the slopes of m, n, p and q using the slope formula
m =
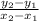
• Parallel lines have equal slopes
• The product of the slopes of perpendicular lines = - 1
For line m
using (x₁, y₁ ) = (- 2, 7) and (x₂, y₂ ) = (3, 5) ← 2 points on the line
m =
 = -
= -

For line n
using (x₁, x₂ ) = (- 5, 0) and (x₂, y₂ ) = (0, - 2) ← 2 points on the line
m =
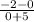 = -
= -

For line p
using (x₁, y₁ ) = (6, 15) and (x₂, y₂ ) = (10, 5) ← 2 points on the line
m =
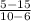 =
=
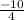 = -
= -
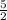
For line q
using (x₁, y₁ ) = (6, 15) and (x₂, y₂ ) = (0, 0) ← 2 points on the line
m =
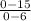 =
=
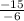 =
=
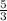
-------------------------------------------------------------------------------------------
Checking the list
m ≠ parallel to q ( slopes are not equal )
q ≠ perpendicular to n ( product of slopes ≠ - 1 )
n ≠ parallel to q ( slopes are not equal )
p ≠ perpendicular to m ( product of slopes ≠ - 1 )
m is parallel to n ( slopes are equal )
p ≠ perpendicular to q ( product of slopes ≠ - 1 )
Thus m is parallel to n ← only one from list