Answer:
3 in
Explanation:
Sum of all sides of ∆ = perimeter
Given that it has a perimeter of 13 in, and the following sides: (x - 5) in, x/2 in, and 6 in, therefore:
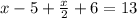
Solve to find the value of x

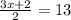
Multiply both sides by 2

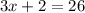
Subtract 2 from both sides
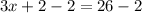
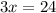
Divide both sides by 3
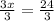
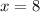
The shortest side = (x - 5) in
Plug in the value of x
= (8 - 5) in
= 3 in