Question

The weight of an organ in adult males has a​ bell-shaped distribution with a mean of grams and a standard deviation of grams. Use the empirical rule to determine the following. ​(a) About ​% of organs will be between what​ weights? ​(b) What percentage of organs weighs between grams and ​grams? ​(c) What percentage of organs weighs less than grams or more than ​grams? ​(d) What percentage of organs weighs between grams and ​grams?

Answer 1

(a) 99.7% of organs will be between 185 grams and 455 grams.

(b) 68% of organs weighs between 275 grams and 365 ​grams.

(c) The percentage of organs weighs less than 275 grams or more than 365 ​grams is 32%.

(d) The percentage of organs weighs between 230 grams and 365 ​grams is 81.5%.

Explanation:

The complete question is: The weight of an organ in adult males has a​ bell-shaped distribution with a mean of 320 grams and a standard deviation of 45 grams. Use the empirical rule to determine the following.​ (a) About 99.7​% of organs will be between what​ weights?​ (b) What percentage of organs weighs between 275 grams and 365 ​grams? ​(c) What percentage of organs weighs less than 275 grams or more than 365 ​grams? ​(d) What percentage of organs weighs between 230 grams and 365 ​grams?

We are given that the weight of an organ in adult males has a​ bell-shaped distribution with a mean of 320 grams and a standard deviation of 45 grams.

Let X = the weight of an organ in adult males

So, X ~ Normal(
`\mu=320, \sigma^(2) =45^(2)`)

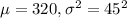The z-score probability distribution for the normal distribution is given by;

Z =
`(X-\mu)/(\sigma)` ~ N(0,1)

where,
`\mu` = population mean weight = 320 grams

`\sigma` = standard deviation = 45 grams

Now, the empirical rule states that;

• 68% of the data values lies within one standard deviation from the mean.

• 95% of the data values lies within two standard deviation from the mean.

• 99.7% of the data values lies within three standard deviation from the mean.

(a) We have to find 99.7% of organs will be between what​ weights;

As we know that 99.7% of the data values lies within three standard deviation from the mean, that means;

`\mu-3\sigma` =
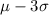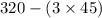`320-(3 * 45)` = 185 grams

`\mu+3\sigma` =
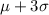`320+(3 * 45)` = 455 grams

Hence, 99.7% of organs will be between 185 grams and 455 grams.

(b) The percentage of organs weighs between 275 grams and 365 ​grams is given by;

z score for 275 grams =
`(X-\mu)/(\sigma)`

=
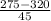`(275-320)/(45)` = -1

z score for 365 grams =
`(X-\mu)/(\sigma)`

=
`(365-320)/(45)` = 1

This means that 68% of organs weighs between 275 grams and 365 ​grams.

(c) The percentage of organs weighs less than 275 grams or more than 365 ​grams is given by;

As we see in the above part that 68% of organs weighs between 275 grams and 365 ​grams, this means that the percentage of organs weighs less than 275 grams or more than 365 ​grams will be = 1 - 68% = 0.32 or 32%.

So, the percentage of organs weighs less than 275 grams =
`(32\%)/(2)` = 16%

and the percentage of organs weighs more than 365 grams =
`(32\%)/(2)` = 16%.

(d) The percentage of organs weighs between 230 grams and 365 ​grams is given by;

z score for 230 grams =
`(X-\mu)/(\sigma)`

=
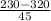`(230-320)/(45)` = -2

z score for 365 grams =
`(X-\mu)/(\sigma)`

=
`(365-320)/(45)` = 1

As we know that 95% of the data values lies within two standard deviation from the mean, that means 5% of the data lies outside 2 standard deviations.

So, the percentage of organs weight between 230 grams and the mean =
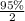`(95\%)/(2)` = 47.5%

Similarly, 68% of the data values lies within one standard deviation from the mean, that means 32% of the data lies outside one standard deviation.

So, the percentage of organs weight between mean and 365 grams =
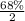`(68\%)/(2)` = 34%

Hence, the percentage of organs weighs between 230 grams and 365 ​grams = 47.5% + 34% = 81.5%.