Answer:
6.93 × 10⁶ s
Step-by-step explanation:
We know that the rate of gas leakage in the balloon is given by
dm/dt = -kCV where m = mass of gas, k = mass transfer coefficient = 1 × 10⁻⁷ cm/s, C = concentration of gas in balloon and V = volume of gas in balloon.
We know m = CV, so
dm/dt = dCV/dt = -kCV
CdV/dt = -kCV
dV/dt = -kV
Separating the variables, we have
dV/V = -kdt
Integrating both sides, we have
∫dV/V = -∫kdt
㏑V = -kt + C
V = exp(-kt)exp(C)
V =
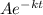
when t = 0, V = V₀
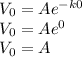

Now since V₀ = 1000 cm³ and we require the time when V = 500 cm³.
Substituting V and V₀ into the equation, we have
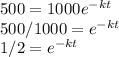
taking natural logarithm of both sides, we have
㏑(1/2) = -kt
t = -㏑(1/2)/k
substituting the value of k we have
t = -㏑(1/2)/1 × 10⁻⁷ cm/s
t = 0.693 × 10⁷ s
t = 6.93 × 10⁶ s