Question

When baseball players throw the ball from the out eld, they usually allow it to takeone bounce before it reaches the in eld because they think that the ball will arrive sooner that way.Suppose that the angle at which a bounced ball leaves the ground is the same as the angle at whichit is thrown but the ball's speed after the bounce is one-half of what it was just before the bounce.(a) Assume that the ball is always thrown with the same initial speed. At what angleshould the elder throw the ball to make it go the same distanceDwith one bounce as a ball thrown upwardsat 45with no bounce

Answer 1

Complete Question

The diagram illustrating this question is shown on the first uploaded image

Answer:

The value is
`\theta = 27^o`

Step-by-step explanation:

Generally the distance covered by the ball thrown at angle 45° that did not bounce is mathematically represented as

`D = v cos (45) * t`

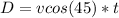
Here t is the total time duration which is mathematically represented as

`t = (v sin (45))/(g)`

This distance distance is also mathematically represented as

`D = vcos(\theta)* t_1 + (v)/(2) cos(\theta )*t_2`

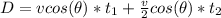
Here
`\theta` the angles made as shown in the diagram

Here
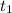`t_1` is the time before the first bounce which is mathematically represented as

`t_1 = (v sin (\theta ))/(g)`

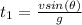
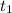`t_1` is the time duration before the final point which is mathematically represented as

`t_2 = ((v)/(2) * sin (\theta ))/(g)`

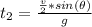
So

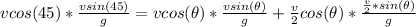
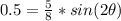
`v cos (45) * (v sin (45))/(g) = vcos(\theta)* (v sin (\theta ))/(g) + (v)/(2) cos(\theta ) * ((v)/(2) * sin (\theta ))/(g)`

=>
`cos (45) sin(45) = cos(\theta)sin(\theta ) + cos(\theta )sin((\theta)/(4))`

=>
`0.5 = (5)/(8) * sin(2\theta )`

=>
`\theta = 27^o`