Answer:
26 yards
Explanation:
The complete question is attached in the image below.
The distance between two points O(
 ) and P(
) and P(
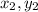 ) is:
) is:
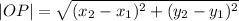
The location of the points of the polygon are as follows:
A(-5, 5), B(0. 5), C(4,2), D(1, -2), E(-2, -2), F(-5, 2). Therefore:

The perimeter of the fence = |AB| + |BC| + |CD| + |DE| + |EF| + |FA| = 5 + 5 + 5 + 3 + 5 + 3 = 26 yards.