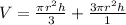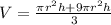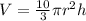Question

A local company makes cement posts in the shape of the following three-dimensional

composite

The cone and cylinder both have the same radius, while the height of the cylinder is 3 times the height of the cone.

Which equation can be used to find the total volume of the cement post?

V= 4pi(r^2)h
V= 4pi(r^4)h
V= 10/3pi(r^2)h
V= 8/3pi(^2)h

Answer 1

`V = (10)/(3) \pi r^2h`

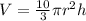
Explanation:

The cone has the following given dimensions:

Radius = r

Height = h

Volume of a cone = ⅓πr²h

The Cylinder has:

Radius = r

Height = 3h

Volume of the cylinder = πr²h = πr²*3h = 3πr²h

Volume of the cement post = volume of Cone + volume of Cylinder

Equation to find total volume of cement post:

V = ⅓πr²h + 3πr²h

`V = (\pi r^2h)/(3) + (3 \pi r^2h)/(1)`

`V = (1(\pi r^2h) + 3(3 \pi r^2h))/(3)`

`V = (\pi r^2h + 9 \pi r^2h)/(3)`

`V = (10 \pi r^2h)/(3)`

`V = (10)/(3) \pi r^2h`