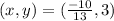Question

Find the coordinates of the point

3

10

of the way from A to B.

AY

10-

B(10,7)

6-

4-

2

X

-6 -4

-2

-22

2

4

6

8

10 12 14

-4-

НА

(-4,-6)

-8-

Answer 1

`(x,y) = ((-10)/(13),3)`

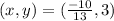
Explanation:

Question is not well formatted; However, the given parameters are

Given

Point = 3/10

A = (-4,-6)

B = (10,7)

Required

Determine the coordinates of the point

Represent the point as ratio

`Point = 3:10`

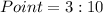
The coordinates can be solved using

`(x,y) = ((mx_2 + nx_1)/(m+n),(my_2 + ny_1)/(m+n))`

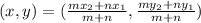
Where

`m:n = 3:10`

`(x_1,y_1) = (-4,-6)`

`(x_2,y_2) = (10,7)`

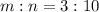
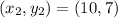
Substitute these values in the above formula:

`(x,y) = ((mx_2 + nx_1)/(m+n),(my_2 + ny_1)/(m+n))`

`(x,y) = ((3 * 10 + 10 * -4)/(3+10),(3 * 7 + 10 * -6)/(10+3))`

`(x,y) = ((30 -40)/(13),(21 -60)/(13))`

`(x,y) = ((-10)/(13),(-39)/(13))`

`(x,y) = ((-10)/(13),3)`

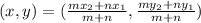
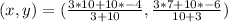
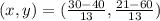
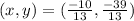
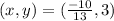
Hence;

The coordinates of the point is
`(x,y) = ((-10)/(13),3)`