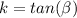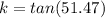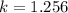Question

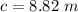
In a certain right triangle, the two sides that are perpendicular to each other are 6.9 m and 5.5 m long. What is the length of the third side? Answer in units of m. What is the tangent of the angle for which 6.9 m is the opposite side?

Answer 1

The length of the third side is

`c =8.82 \  m`

The tangent of the angle for which 6.9 m is the opposite side is

`k = 1.256`

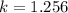
Step-by-step explanation:

From the question we are told that

The first side is a = 6.9 m

The second side is b = 5.5 m

Generally apply Pythagoras theorem

`c^2 = a^2 + b^2`

=>
`c = √(a^2 + b^2 )`

=>
`c = √(6.9^2 + 5.5^2 )`

=>
`c =8.82 \ m`

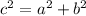
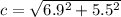
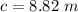
From sin rule we have that

`(c)/(sin(\theta )) = (a)/(sin (\beta ))`

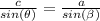
Generally from a right triangle the angle
`\theta = 90`

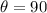
So

`(8.82)/(sin(90 )) = (6.9)/(sin (\beta ))`

=>
`\beta = sin ^(-1)[(6.9)/(8.82) ]`

=>
`\beta =51.47^o`

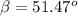
Generally the tangent of the angle for which 6.9 m is the opposite side is mathematically represented as

`k = tan (\beta )`

`k  =  tan (51.47 )`

`k  = 1.256`