Question

Lindsey Wilson was the top scorer in a women's professional basketball league for the 2006 regular season, with a total of 814 points. The number of two-point field

goals that Lindsey made was 49 less than double the number of three-point field goals she made. The number of free throws (each worth one point) she made was

38 less than the number of two-point field goals she made. Find how many free throws, two-point field goals, and three-point field goals Lindsey Wilson made during

the 2006 regular season.

Lindsey Wilson made free throws.

Answer 1

The number of free throws, two-point throw, and three-point throw are 135, 173, and 111 respectively.

Explanation:

Let x,y, and z are the numbers of two-point field goals, numbers of three-point field goals, and the number of free-throws (one-point goal) respectively.

The total points= 814

`\Rightarrow 2x+3y+z=814\;\cdots(i)`

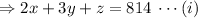
As the number of two-point goals was 49 less than double the number of three-point field goals she made.

`\Rightarrow x=2y-49 \;\cdots(ii)`

Again, the number of free goals was 38 less than the number of two-point field goals she made.

`\Rightarrow z=x-38\;\cdots(iii)`

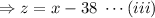
From equations (i) and (iii)

2x+3y+x-38=814

`\Rightarrow 3x+3y=852`

`\Rightarrow x+y=284`

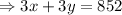
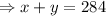
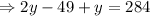`\Rightarrow 2y-49+y=284` [using equation (ii)]

`\Rightarrow 3y=333`

`\Rightarrow y=111`

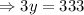
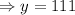
From equation (ii),

`x= 2*111-38=173`

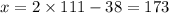
From equation (iii),

`z=173-38=135`.

Hence,

The number of free throws, z= 135

The number of two-point throw, x=173

The number of three-point throw, y=111.