Question

Find limit. Please answer ​

Answer 1

`\displaystyle \lim_(x \to 2) (x^8 - 256)/(x - 2) = 1024`

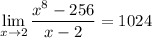
General Formulas and Concepts:

Calculus

Limits

Limit Rule [Constant]:
`\displaystyle \lim_(x \to c) b = b`

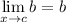
Limit Rule [Variable Direct Substitution]:
`\displaystyle \lim_(x \to c) x = c`

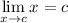
Limit Property [Addition/Subtraction]:
`\displaystyle \lim_(x \to c) [f(x) \pm g(x)] = \lim_(x \to c) f(x) \pm \lim_(x \to c) g(x)`

L'Hopital's Rule

Differentiation

• Derivatives
• Derivative Notation

Basic Power Rule:

1. f(x) = cxⁿ
2. f’(x) = c·nxⁿ⁻¹

Explanation:

We are given the following limit:

`\displaystyle \lim_(x \to 2) (x^8 - 256)/(x - 2)`

Substituting in x = 2 using the limit rule, we have:

`\displaystyle \lim_(x \to 2) (x^8 - 256)/(x - 2) = (2^8 - 256)/(2 - 2)`

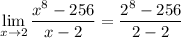
Evaluating the result, we get an indeterminate form:

`\displaystyle \lim_(x \to 2) (x^8 - 256)/(x - 2) = (0)/(0)`

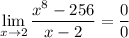
Since we have an indeterminate form, let's apply L'Hopital's Rule. Differentiate both the numerator and denominator respectively:

`\displaystyle \lim_(x \to 2) (x^8 - 256)/(x - 2) = \lim_(x \to 2) (8x^7)/(1)`

Simplify:

`\displaystyle \lim_(x \to 2) (x^8 - 256)/(x - 2) = \lim_(x \to 2) 8x^7`

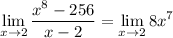
Evaluate the limit using the limit rule:

`\displaystyle \lim_(x \to 2) 8x^7 = 8(2)^7`

Simplify:

`\displaystyle \lim_(x \to 2) 8x^7 = 1024`

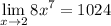
And we have our answer.

Topic: AP Calculus AB/BC (Calculus I/I + II)

Unit: Limits