Answer:
If this solution is under standard conditions, then it will be possible to explain the products of this electrolysis (
 ,
,
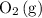 ,
,
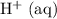 ) with reference to the standard reduction potentials.
) with reference to the standard reduction potentials.
Step-by-step explanation:
Assume that this solution is under standard conditions, including:
- A concentration of
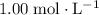 ,
, - A pressure of
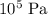 , and
, and - A temperature of
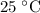 .
.
This
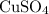 solution (in water) would include the following species:
solution (in water) would include the following species:
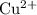 ions,
ions,
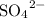 ions, and
ions, and
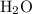 molecules.
molecules.
(The carbon electrode is relatively inert and typically won't take part in the chemical reaction.)
Consider the electrolysis of this solution as the sum of the reduction half-reaction and the oxidation half-reaction. Look up the standard reduction potential for half-reactions involving these species:
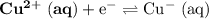 . Standard reduction potential:
. Standard reduction potential:
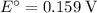 .
.
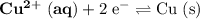 . Standard reduction potential:
. Standard reduction potential:
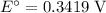 .
.
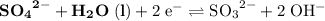 . Standard reduction potential:
. Standard reduction potential:
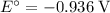 .
.
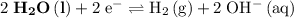 . Standard reduction potential:
. Standard reduction potential:
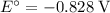 .
.
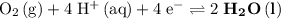 . Standard reduction potential:
. Standard reduction potential:
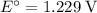 .
.
(Species found in this solution are shown in bold typeface.)
Reduction Half-reaction
Note, that in the first four reactions, species from this solution are all on the same side as
 . In other words, in those four reactions, these species would gain electrons and are reduced. These reactions are thus plausible reduction half-reactions. The standard electrode potential of each half reaction will be the same as the standard reduction potential. Because reduction takes place at the cathode, these potentials are denoted as
. In other words, in those four reactions, these species would gain electrons and are reduced. These reactions are thus plausible reduction half-reactions. The standard electrode potential of each half reaction will be the same as the standard reduction potential. Because reduction takes place at the cathode, these potentials are denoted as
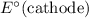 .
.
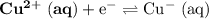 .
.
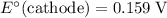 .
.
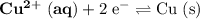 .
.
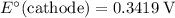 .
.
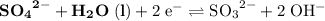 .
.
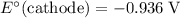 .
.
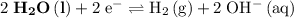 .
.
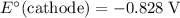 .
.
The only reduction half-reaction that will take place will be the one with the most positive (least negative) electrode potential. Among these four plausible half-reaction, that reaction would be the reduction of
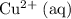 to
to
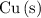 (elemental copper,)
(elemental copper,)
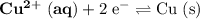 , with an electrode potential of
, with an electrode potential of
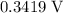 . That explains why metallic copper will deposit on the cathode.
. That explains why metallic copper will deposit on the cathode.
Oxidation Half-reaction
On the other hand, the reaction
 seems to be the only one that involves species from this solution on the opposite side of
seems to be the only one that involves species from this solution on the opposite side of
 . The actual reaction would take place in the other direction:
. The actual reaction would take place in the other direction:
 .
.
The corresponding electrode potential will be the opposite of the standard reduction potential. Because this reaction corresponds to oxidation, it will happen at the anode.
 .
.
That explains why
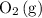 will be produced at the anode.
will be produced at the anode.
Overall Reaction
Multiply coefficients in the reduction half-reaction by two and combine the two half-reactions. Make sure that electrons are eliminated from both sides of this equation:
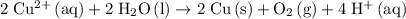 .
.
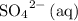 would be the spectator ion. Add that to the reaction to obtain the chemical equation of this electrolysis:
would be the spectator ion. Add that to the reaction to obtain the chemical equation of this electrolysis:
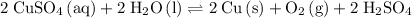 .
.