Question

Find the smallest number by which 240 must be multiplied so that the product is a perfect square.

Answer 1

`\large{\underline{\underline{\red{\tt{\purple{\leadsto } SolutioN:-}}}}}`

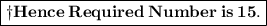
Here we are asked to find the smallest number which must be multiplied to 240 to make it a perfect square .

So , lets do prime factorisation of 240 .

Here , 240 = 2 × 120 .

= 2 × 2 × 60.

= 2 × 2 × 2 × 30.

= 2 × 2 × 2 × 2 × 3 × 5 .

Hence we got 240 = 2⁴ × 3¹ × 5¹ .

Now here we can see that , in order to make a perfect square it should be multiplied by 3 × 5 = 15 in order to make power of prime factors even .

`\boxed{\green{\bf{\dag Hence\: Required\: Number\:is\:15.}}}`