Question

If alpha and beta are the zeroes of the polynomial f(x)= xsquare -8x +gamma such that alpha - beta = 2 find the value of gamma. Hint( alpha-beta=2 then alpha-beta whole square is 4. Help please..​❤would mean a lot.

xx

Answer 1

Γ = 15

Explanation:

Given

f(x) = x² - 8x + Γ

with a = 1, b = - 8 and c = Γ , then

sum of zeros α + β = -
`(b)/(a)` = -
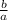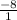`(-8)/(1)` = 8

product of zeros = αβ =
`(c)/(a)` = Γ

Given α - β = 2 , then

(α - β)² = 2²

α² - 2αβ + β² = 4 → (1)

and

(α + β)² = 8²

α² + 2αβ + β² = 64 → (2)

Add (1) and (2) term by term

2α² + 2β² = 68 ( divide through by 2 )

α² +β² = 34

Substitute α² + β² = 34 into (1)

34 - 2αβ = 4 ( subtract 34 from both sides )

- 2αβ = - 30 ( divide both sides by - 2 )

αβ = 15

Now

αβ = Γ = 15

Thus

f(x) = x² - 8x + 15