Answer:
Amount of fencing required = 75 yards
Explanation:
Distance between the two points
 and
and
 is given by the formula,
is given by the formula,
d =
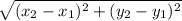
Distance between A(3, 6) and B(3, -2) =
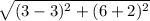
= 8 yards
Distance between B(3, -2) and C(-7, 4) =
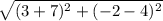
=
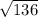
= 11.66 ≈ 12 yards
Distance between C(-7, 4) and D(-7, -2) =
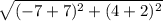
= 6 yards
Distance between D(-7, -2) and E(-3, -2) =
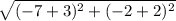
= 4 yards
Distance between E(-3, -2) and F(-3, -8) =
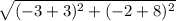
= 6 yards
Distance between F(-3, -8) and G(3, -8) =
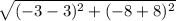
= 6 yards
Distance between G(3, -8) and H(10, -12) =
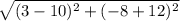
=

=
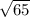
= 8.06 ≈ 8 yards
Distance between H(10, -12) and J(10, 6) =
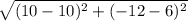
= 18 yards
Distance between A(3, 6) and J(10, 6) =
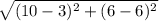
= 7 yards
Since length of fence required = perimeter of the flat area
Perimeter of the given area = 8 + 12 + 6 + 4 + 6 + 6 + 8 + 18 + 7
= 75 yards
Therefore, amount of fencing required = 75 yards