Answer:
Approximately
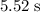 (assuming that
(assuming that
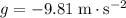 .)
.)
Step-by-step explanation:
From the perspective of an observer on the ground, the package was initially moving with the helicopter. The initial velocity of that package (
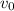 ) would be the same as the velocity of the helicopter. In other words,
) would be the same as the velocity of the helicopter. In other words,
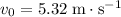 .
.
Other quantities:
 . (The
. (The
 here is negative because the acceleration due to gravity points downwards.)
here is negative because the acceleration due to gravity points downwards.)
 (The position of the package would have changed by
(The position of the package would have changed by
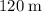 by the time it reached the ground. The negative sign is because this position change also points downwards.)
by the time it reached the ground. The negative sign is because this position change also points downwards.)
There are two ways to find the time
 required for the package to reach the ground after it was dropped.
required for the package to reach the ground after it was dropped.
The first approach makes use of the SUVAT equation:
 .
.
For this question,
 ,
,
 , and
, and
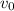 are already known. However, this approach would require solving a quadratic equation where the coefficient for the first-order term is non-zero.
are already known. However, this approach would require solving a quadratic equation where the coefficient for the first-order term is non-zero.
An alternative approach is to solve for
 , the velocity of the package right before it reaches the ground. From the SUVAT equation that does involve time:
, the velocity of the package right before it reaches the ground. From the SUVAT equation that does involve time:
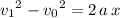 .
.
Again,
 ,
,
 , and
, and
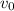 are already known. Solve for
are already known. Solve for
 :
:
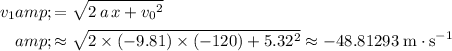 .
.
Calculate time from the change in velocity:
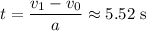 .
.