Question

Teresa runs 7 miles in 80 minutes. At the same rate, how many miles would she run in 64 minutes?

Answer 1

She would run about 5.8 miles in 64 min.

Explanation:

Let m= miles per minute

7=80m divide 80 on both sides.

0.0875=m Round

0.09 miles per minute

0.09 x 64= 5.76 round or leave it like that

5.8

Answer 2

`\boxed {\tt 5.6 \ miles}`

Explanation:

Let's set up a proportion using the following setup:

`(miles)/(minutes)= (miles)/(minutes)`

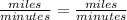
We know Teresa can run 7 miles in 80 minutes.

`(7 \ miles)/(80 \ minutes)=(miles)/(minutes)`

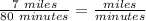
We don't know how many miles she can run in 64 minutes. Therefore, she can run x miles in 64 minutes.

`(7 \ miles)/(80 \ minutes)=(x \ miles)/(64 \ minutes)`

`(7)/(80) =(x)/(64)`

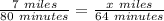
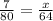
We want to solve for x ( miles in 64 minutes). We must isolate x on one side of the proportion. x is being divided by 64 and the inverse of division is multiplication. Multiply both sides of the equation by 64.

`64*(7)/(80) =(x)/(64)*64`

`64*(7)/(80) =x`

`64*0.0875=x`

`5.6=x`

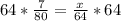
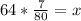
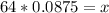
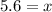
x= 5.6 miles

Teresa can run 5.6 miles in 64 minutes.