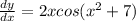Question

If y=sin(x²+7) find Dy/Dx​

Answer 1

Answer dy/dx = 2x cos (x to the second power + 7)

Explanation:

Answer 2

`(dy)/(dx) = 2xcos(x^2+7)`

Explanation:

Given:
`y=sin(x^2+7)`

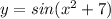
Find:
`(dy)/(dx)`

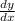
For this problem, it looks like we are going to use the chain rule. We have to functions, let's label them:

`f(x)=sin(x)\\g(x)=x^2+7`

Let's remember that the chain rule is:
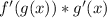`f'(g(x))*g'(x)` or
`((dy)/(du) )((du)/(dx) )`

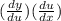
Since these functions are basic, I will be using the
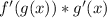`f'(g(x))*g'(x)` rule, but both rules always work. We need to find
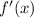`f'(x)` and
`g'(x)`

`f'(x)=cos(x)\\g'(x)=2x`

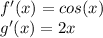
Remember that the derivative of
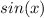`sin(x)` is
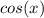`cos(x)`. Make sure you know all your trig derivatives. Now that we know all our variables, we can plug them in. We will take
`g(x)` and plug it into
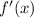`f'(x)` and multiply it all by
`g'(x)`.

`[cos(x^2+7)][2x]=2xcos(x^2+7)`

Since nothing else can be done to reduce this expression, your answer is:

`(dy)/(dx) = 2xcos(x^2+7)`