Question

I got 8x/3cubroot of (1+8x). Is this right can someone explain the steps that they did to get the answer?

Answer 1

`(dy)/(dx)[\sqrt[3]{1+8x} ] = \frac{8}{3\sqrt[3]{(1+8x)^2} }`

Step-by-step explanation:

We need to use the chain rule, and you correctly identified it; however, you did not apply it correctly. Let's review it.

The chain rule is
`f'(g(x)) * g'(x)`

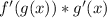
To solve, we need
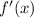`f'(x)` and
`g'(x)`. Let's find them.

`f'(x)= (d)/(du) [\sqrt[3]{u} ] = (d)/(du) [u^(1)/(3) ] = (1)/(3)u^(-2)/(3) = (u^(-2)/(3) )/(3) = (1)/(3u^(2)/(3) ) \\g'(x)= (d)/(dx) [1+8x] = 8`

For both these derivatives, I used the power rule. For
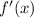`f'(x)`, I just simplified it completely to make it look nicer. Now that we know everything, we need to plug it into the chain rule:
`f'(g(x)) * g'(x)`

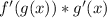
Take the
`g(x)` function and put it into
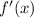`f'(x)` and multiply all that by
`g'(x)`.

`((1)/(3(1+8x)^(2)/(3) ) )((8)/(1) ) = (8)/(3(1+8x)^(2)/(3) ) = \frac{8}{3\sqrt[3]{(1+8x)^2} }`

So, your derivative is
`\frac{8}{3\sqrt[3]{(1+8x)^2} }`