Answer:
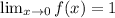
Explanation:
So we have the graph of f(x).
And we want to determine the following:
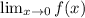
To do so, look at the graph and see the value f(x) is approaching as x approaches 0.
We can see that as x gets closer and closer to 0, f(x), or the y-value, gets closer and closer to 1.
In other words, our answer is:
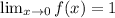
Edit: Typo.