Answer:
The % of drivers that will exceed this limit is 10.56 %
Explanation:
Let's start defining the random variable :
 : '' The speed on that road ''
: '' The speed on that road ''
We know that
 can be modeled with a Gaussian distribution ⇒
can be modeled with a Gaussian distribution ⇒
 ~
~
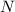 ( μ , σ )
( μ , σ )
Where ''μ'' is the mean and ''σ'' is the standard deviation. Given that the average speed and the standard deviation of the problem are known we write :
 ~
~
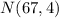
We are asked about
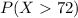 (which is the % of drivers that will exceed this limit).
(which is the % of drivers that will exceed this limit).
To find this probability we are going to make a standardization of the variable
 (also called a change of variables).
(also called a change of variables).
We are going to substract the mean to
 and then divide by its standard deviation :
and then divide by its standard deviation :
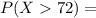 P [(X-μ) / σ > (72 - μ) / σ] (I)
P [(X-μ) / σ > (72 - μ) / σ] (I)
The new variable [(X - μ) / σ] is called Z.
Z can be modeled as
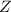 ~
~

⇒ Replacing in (I) the values of the mean and the standard deviation :
 =
=

The convenience of this is that we can find the probabilities of Z (which is a N(0,1) ) in any table on internet ⇒
Looking at any table we will find that
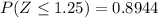 ⇒
⇒
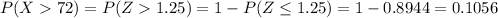 = 10.56 %
= 10.56 %
We find that the % of drivers that will exceed this limits is 10.56 %