Answer:
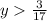
Explanation:
To solve this problem, simply set the binomial,
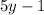 as greater than
as greater than
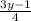 , and solve for the value of y as you would solve an equation.
, and solve for the value of y as you would solve an equation.
Thus:
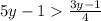
Multiply both sides by 4

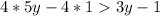
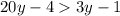
Subtract 3y from both sides

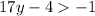
Add 4 to both sides
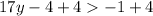
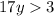
Divide both sides by 17

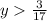
Therefore, the value of y that will make the binomial
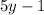 greater than
greater than
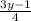 is
is
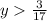 .
.