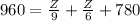Complete Question
A bee flies at 9 feet per second directly to a flowerbed from its hive. The bee stays at the flowerbed for 13 minutes, and then flies directly back to the hive at 6 feet per second. It is away from the hive for a total of 16 minutes. What equation can you use to find the distance of the flowerbed from the hive?
Answer:
The equation is
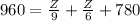
Explanation:
From the question we are told that
The velocity of the bee is

The duration on the flowerbed is
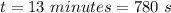
The velocity back to his hive is
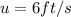
The total time away from it hive is
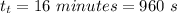
Now let assume the distance of the flower bed from the hive is Z
Generally the time taken for the bee to travel to the flower bed from its hive is

The time taken for the bee to fly back to his hive is
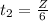
Generally the total time the bee spent outside of his house is mathematically represented as
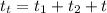
=>