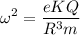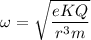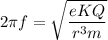Question

You are part of a design team assigned the task of making an electronic oscillator that will be the timing mechanism of a micro-machine. You start by trying to understand a simple model which is an electron moving along an axis through the center and perpendicular to the plane of a thin positively charged ring. You need to determine how the oscillation frequency of the electron depends on the size and charge of the ring for displacements of the electron from the center of the ring along the axis that are very small compared to the size of the ring. A team member suggests that you first determine the acceleration of the electron along the axis as a function of the size and charge of the ring and then use that expression to determine the oscillation frequency of the electron for small oscillations.Express your answer for the oscillation frequency in terms of the mass (m) and charge (e) of the electron, the charge (q) and radius (r) of the ring, and Coulomb's constant (k). (All letters are lowercase, remember that "e" is a positive constant.)

Answer 1

We assume that there is a ring having a charge +Q and radius r. Electric field due to the ring at a point P on the axis is given by :

`E_P=\int dE \cos`

`E_P=\int (KdQ)/((√(r^2+x^2))^2)* (x)/(√(r^2+x^2))`

`\vec{E_P}=(Kx)/(r^2+x^2) \int dQ`

`\vec{E_P}=(KxQ)/((r^2+x^2)^(3/2)) \hat{i}`

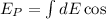
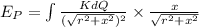
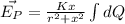
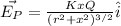
If we put an electron on point P, then force on point e is :

`\vec{F}=-|e|\vec{E_P}`

`F= (-eKQx)/((r^2+x^2)^(3/2))= (-eKQx)/(r^3[1+(x^2)/(r^2)]^(3/2))`

If r >> x , then
`$(x^2)/(r^2) \approx 0$`

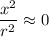
Then,
`$(-eKQ)/(r^3)x$`

`$ma =(-eKQ)/(r^3)x$`

`$a =(-eKQ)/(mr^3)x$`

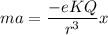
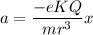
Compare, a = -ω²x

We get,

`$\omega^2 = (eKQ)/(R^3m)$`

`$\omega = \sqrt{(eKQ)/(r^3m)}$`

`$2 \pi f = \sqrt{(eKQ)/(r^3m)}$`

`$f = (1)/(2 \pi)\sqrt{(eKQ)/(mr^3)}$`