Answer:
The calculated t- value = 1.11 > 2.89 at 0.025 level of significance
Null hypothesis is rejected
The engineer designed the valve such that it would produce a mean pressure is not equal to 5.4
Explanation:
Step(i):-
Given mean of the Population (μ) = 5.4
Given sample size 'n' = 9
Mean of the sample (x⁻) = 5.7
Standard deviation of the sample (s) = 0.81
Step(ii):-
Null Hypothesis: H₀: The engineer designed the valve such that it would produce a mean pressure of 5.4
H₀: μ = 5.4
Alternative Hypothesis : H₁: μ ≠ 5.4
Level of significance = 0.025
Test statistic
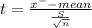
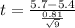
t = 1.11
Degrees of freedom
ν = n-1 = 9-1 =8
t₀.₀₁₅ , ₈ = 2.89
The calculated t- value = 1.11 > 2.89 at 0.025 level of significance
Null hypothesis is rejected
The engineer designed the valve such that it would produce a mean pressure is not equal to 5.4