Question

pls I need help really quickly sooo if you see this , help, "Ashley has a right triangular garden in her backyard. The longest side of the garden measures 17 yards. One of the sides is 10 yards long. Find the length of the other side of the garden. (round to the nearest hundredths)"

Answer 1

`\boxed {\tt 13.75 \ yards}`

Explanation:

Since this is a right triangle, we can use the Pythagorean Theorem.

`a^2+b^2=c^2`

where
`a` and
`b` are the legs and
`c` is the hypotenuse.

One of the legs is 10 yards, and the other is unknown. The hypotenuse is 17 yards, because it is the longest side.

`a= 10 \ yd\\b=b\\c= 17 \ yd`

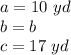
Substitute the values into the formula.

`(10 \ yd)^2+b^2=(17 \ yd)^2`

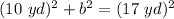
Evaluate the exponents.

• (10 yd)²= 10 yd * 10 yd = 100 yd²

`100 \ yd^2+b^2= (17 \ yd)^2`

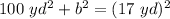
• (17 yd)²= 17 yd * 17 yd=289 yd²

`100 \ yd^2+b^2= 289 \ yd^2`

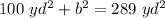
Now, solve for b. First, subtract 100 yards squared from both sides of the equation.

`100 \ yd^2-100 \ yd^2+b^2= 289 \ yd^2- 100 \ yd^2`

`b^2=289\ yd^2-100 \ yd^2`

`b^2=189 \ yd^2`

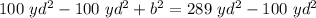
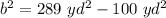
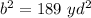
Finally, take the square root of each side of the equation.

`√(b^2) =√(189\ yd^2)`

`b=√(189 \ yd^2)`

`b=13.7477271 \ yd`

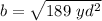
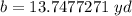
Round to the nearest hundredth. The 7 in the thousandth place tells us to round the 4 to a 5.

`b \approx 13.75 \ yd`

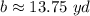
The other side of the garden is about 13.75 yards long.