Question

How do you solve this? Step by step please

2^x=5e^(x+1)

Answer 1

`x=(\ln(5)+1)/(\ln(2)-1)\approx-8.5039`

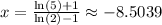
Explanation:

So we have the equation:

`2^x=5e^(x+1)`

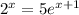
Let's take the natural log of both sides:

`\ln(2^x)=\ln(5e^(x+1))`

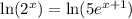
On the left, we can move the x to the front:

`x\ln(2)=\ln(5e^(x+1))`

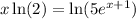
On the right, we can separate the logs:

`x\ln(2)=\ln(5)+\ln(e^(x+1))`

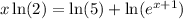
For the second term on the right, move all the exponent stuff to the front:

`x\ln(2)=\ln(5)+(x+1)\ln(e)`

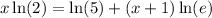
The natural log of e is 1. So:

`x\ln(2)=\ln(5)+(x+1)(1)`

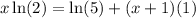
Simplify:

`x\ln(2)=\ln(5)+x+1`

Subtract x from both sides:

`x\ln(2)-x=\ln(5)+1`

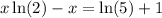
Factor out an x from the left:

`x(\ln(2)-1)=\ln(5)+1`

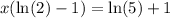
Divide both sides by the equation in the factor:

`x=(\ln(5)+1)/(\ln(2)-1)`

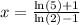
So, our answer is:

`x=(\ln(5)+1)/(\ln(2)-1)\approx-8.5039`

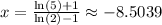
And we're done!