Answer:
The absolute maximum is
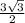 and the absolute minimum value is
and the absolute minimum value is

Explanation:
Differentiate of
 both sides w.r.t.
both sides w.r.t.
 ,
,
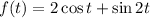
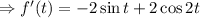
Now take
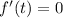


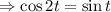
![\Rightarrow 1-2\sin ^2t =\sin t \quad \quad [\because \cos 2t = 1-2\sin ^2t]](https://img.qammunity.org/2021/formulas/mathematics/college/bvmgvwbpm2m37w0uzcie0ycagu57g7wbf3.png)
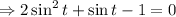
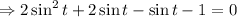
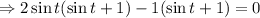
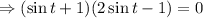
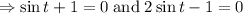
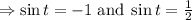
In the interval
 , the answer to this problem is
, the answer to this problem is

Now find the second derivative of
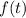 w.r.t.
w.r.t.
 ,
,
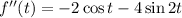
![\Rightarrow \left[f''(t)\right]_{t=\frac {\pi}6}=-2* \frac {\sqrt 3}2-4* \frac{\sqrt 3}2=-3\sqrt 3](https://img.qammunity.org/2021/formulas/mathematics/college/yko2cwr6fwa8ou5hp44x56elbsm4g0hc4a.png)
Thus,
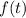 is maximum at
is maximum at
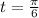 and minimum at
and minimum at
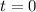
![\left[f(t)\right]_{t=\frac {\pi}6}=2* \frac {\sqrt 3}2+\frac{\sqrt 3}2=\frac{3\sqrt 3}2\;\text{and}\;\left[f(t)\right]_{t=\frac{\pi}2}= 2* 0+0=0](https://img.qammunity.org/2021/formulas/mathematics/college/ncr58el67di81yai54rxkx2f6fdtkju6r2.png)
Hence, the absolute maximum is
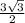 and the absolute minimum value is
and the absolute minimum value is
 .
.