Question

What is the height of the building to the nearest tenth of a foot? (This is timed so help me ASAP please)

Answer 1

Height of the building = 18.3 ft

Explanation:

Let the height of the building = x ft

From the picture attached,

A boy is standing on the building at a point A, car is at a point B and a bird is flying at C.

Applying Pythagoras theorem in ΔABD to find the distance AD.

AB² = BD² + AD²

AD =
`√(AB^2-BD^2)`

=
`√(25^2-20^2)`

=
`√(225)`

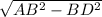
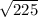
= 15 units

Now from ΔACD,

tan(70)° =
`\frac{\text{CD}}{\text{AD}}`

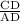
1.22196 =
`(x)/(15)`

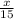
x = 15 × 1.22196

x = 18.33

≈ 18.3 ft

Therefore, height of the building is 18.3 ft.