Answer:
Cylinder

Given:
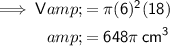
Cube

Given:
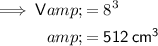
Volume available to be filled with water
Volume of cylinder - volume of cube
= 684π - 512
= 1532.75204 cm³
1 litre = 1000 cm³
⇒ 1.5 litres = 1000 × 1.5 = 1500 cm³
As 1500 < 1532.75204, the volume of water poured into the container is smaller than the empty space available in the cylinder. Therefore, the water will not come over the top of the container.