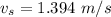Answer:
The value is
Step-by-step explanation:
From the question we are told that
The frequency of the second player is
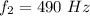
The beat frequency is
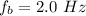
The speed of sound is
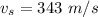
Generally the frequency of the note played by the first player is mathematically represented as
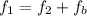
=>
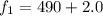
=>
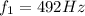
From the relation of Doppler Shift we have that
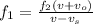
Here

So
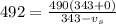
=>