Question

A tensile test uses a test specimen that has a gage length of 50 mm and an area = 206 mm2. During the test, the specimen yields under a load of 97,944 N. The corresponding gage length = 50.2 mm - this is at the 0.2 percent yield point. The maximum load of 162,699 N is reached at a gage length = 63 mm. If fracture occurs at a gage length of 71 mm, determine the percent elongation in % - enter your answer as a whole number, not as a fraction.

Answer 1

The percent elongation in the length of the specimen is 42%

Step-by-step explanation:

Given that:

The gage length of the original test specimen
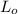`L_o` = 50 mm

The final gage length
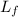`L_f` = 71 mm

The area = 206 mm²

maximum load = 162,699 N

To determine the percent elongation in %, we use the formula:

`\%EL = (L_f-L_o)/(L_o)* 100`

`\%EL = (71 \ mm-50 \ mm)/(50 \ mm)* 100`

`\%EL = (21 mm)/(50 \ mm)* 100`

`\%EL = 0.42 * 100`

`\mathbf{\%EL = 42 \%}`

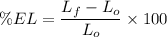
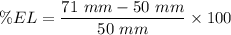
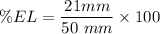
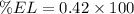
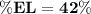
The percent elongation in the length of the specimen is 42%